Take a look at this mnemonic to help your child remember and understand the steps or algorithm for long division. In our house, it is not a good idea to come home late when the family’s favorite dish is served for dinner. However, you can cross your fingers and leave Mom a note. The note may read, “ Dear Mom Save Cheese Burgers.” The first letter of each word represents a step listed below.

Steps
Dear ........ Divide
Mom ......... Multiply
Save ........ Subtract
Cheese .......Check
Burgers ..... Bring Down

The following illustrations will demonstrate step by step how to do long division.


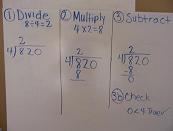Step 1: Divide the divisor, 4, into the first digit of the dividend, 8. Why? We divide eight by four because eight is the first number in the dividend larger than eight. Also, notice the two is written directly above the eight for the same reason.

Step 2: Multiply four times two and write the product directly under the number divided. In this case, it was eight.

Step 3 : Subtract and Check. The purpose of the Check step is to verify the previous steps were done correctly. In order to check, verify that the difference is smaller than the divisor. In this problem, 0 < 4 ? True! So, continue to the next step. If false, check your subtraction and/ or go back to the previous Divide step and check your work from that point.



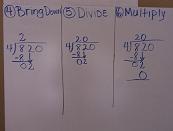Step 4: Bring down the next number in the dividend. Notice the arrow.
The arrow is not required, but it is helpful for the beginner.

Repeat steps from the beginning.

Step 5 Divide: 02 divided by 4 is zero. (Imagine 2 gummy bears. Can you group them into groups of four? No. So, zero is written above the two.) Many students allow this situation to confuse or trick them. It’s okay to get zero for an answer.

Step 6 Multiply: Multiply the divisor by zero. (4 x 0 = 0)

Thereafter, continue to follow the steps.

Let’s look at step 8. When zero was subtracted from two, the answer was two. The answer, two, is smaller than the divisor, four. Therefore, all is well, and we can continue.



























After step 13; notice there is nothing else to bring down.

Step 14 The Last Check: Multiply the quotient (answer) by the dividend. Then add the remainder, if any. The result should equal the dividend. 205 x 4 = 820 which is the same number as the divisor. So, this problem is correct.


Next, let us work a problem that with larger numbers and has a remainder.

649 divided by 15
Study the example below and try to identify each step.



Notice the first digit of the dividend, 6, is smaller than the divisor, fifteen. Thus, fifteen cannot be divided into six. Normally, if the first number in the dividend isn’t large enough, the space above the number is left blank or an “x” is placed above it without going through the remaining steps. Next, place your thumb over the last digit in the dividend, 9, exposing only “64.” Divide 15 into 64 or ask yourself, “fifteen times what number gets me closest to sixty-four without going over?” The answer is 4. Multiply fifteen times four and subtract. When sixty is subtracted from sixty-four, the answer is four. Since four is less than fifteen, we are dividing properly so far. Bring down the next number and repeat the process. Note: Sometimes, it is helpful to round two digit divisors to the nearest ten to make dividing easier and to get a starting point.


To Check: Multiply the quotient (answer) by the divisor. Then add the remainder to the product. The result should equal the dividend. 43 x 45 = 645 + 4 = 649 True; thus the problem is correct.

In order to become comfortable with long division, you must practice. I recommend Richard Fisher’s 80 page workbook, Mastering Essential Math Skills WHOLE NUMBERS AND INTEGERS , from his book series for practice. It is designed for daily practice. The author includes reminders of the steps and answers in the back of the book.